NOTE: WE ARE NOT RESPONSIBLE FOR ANY DAMAGE YOU MAY DO TO YOUR NXT BRICK.
THIS EXERCISE PRESUMES SOME WORKING KNOWLEDGE OF ELECTRONICS.
In this exercise, I will walk you through interfacing a potentiometer (variable resistor) to the NXT brick.
You will need:
– A stripped NXT cable
– A potentiometer with a maximum resistance no more than 
– A small piece of wire
– An NXT Brick
This exercise is derived and expanded from a chapter in Extreme NXT by Gasperi, Hurbain and Hurbain.
THEORY
The NXT monitors the potential difference between the black and white wires with an Analog-to-Digital (A/D) converter. The A/D converter converts this potential difference to a RAW value between 0 and 1023 (10 bits accuracy). This RAW value is given by the ratio
(1) 
where  is the maximum RAW value of 1023,
is the maximum RAW value of 1023,  is the voltage used by the NXT A/D Converter, and
is the voltage used by the NXT A/D Converter, and  is the voltage drop between the black and white wires.
is the voltage drop between the black and white wires.
The circuit diagram looks like this:
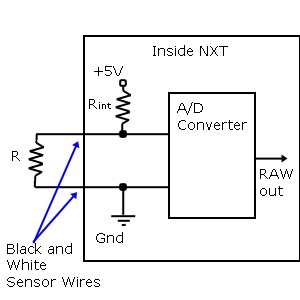
I have a little  potentiometer that can turn over a range of about
potentiometer that can turn over a range of about  to
to  . Below is a diagram. The resistance between the leftmost and rightmost pins is the maximum resistance of
. Below is a diagram. The resistance between the leftmost and rightmost pins is the maximum resistance of  . We will focus on the resistance between the leftmost and center pins, which varies based on the angle through which the potentiometer has been rotated. To keep things safe, we wire the center pin and rightmost pin together. This doesn’t affect the potential difference between the leftmost and center pins.
. We will focus on the resistance between the leftmost and center pins, which varies based on the angle through which the potentiometer has been rotated. To keep things safe, we wire the center pin and rightmost pin together. This doesn’t affect the potential difference between the leftmost and center pins.

I will assume that it is a linear potentiometer (a pretty good assumption), which means that the resistance at any given angle  is given by
is given by
(2) 
where  is the maximum angle of the potentiometer and
is the maximum angle of the potentiometer and  is the
is the  maximum resistance.
maximum resistance.
Equation (2) says that if the angle  then the resistance of the potentiometer
then the resistance of the potentiometer  , and if the angle
, and if the angle  then the resistance of the potentiometer is maximum
then the resistance of the potentiometer is maximum  .
.
Looking at the circuit diagram for the A/D converter, the potential drop across our potentiometer (represented by resistor  ) is given by the typical voltage divider relation
) is given by the typical voltage divider relation
(3) 
We can now substitute (2) into (3) so that the voltage between the black and white wires is determined by the angle of the potentiometer rather than its resistance. Then we can substitute the result into (1) to get an equation for the RAW value
(4) 
with my particular values, this is

This formula will let us predict the NXT RAW value based on the angle of the potentiometer.
For my potentiometer, I find that a maximum angle of  gives me a maximum value of 93. This is less than 7 bits of information, and each RAW value corresponds to
gives me a maximum value of 93. This is less than 7 bits of information, and each RAW value corresponds to  . If you want a nice angle detector, you will probably need a
. If you want a nice angle detector, you will probably need a  potentiometer!
potentiometer!
TRY IT
1. Before beginning, you need to cut and strip one of the NXT cables so that you can interface with the wires directly. I have placed a layer of solder on mine, so they can be inserted into a breadboard for easy connecting.
2. Next connect the center and right pins of the potentiometer together with a wire
3. Plug the other end of the NXT cable into the NXT brick.
I wrote a simple NXT-G program to read the sensor and display the RAW value. Notice that the Touch Sensor actually reads the resistance between the wires. So we are just replacing the Touch Sensor with a potentiometer. We will use the raw number output of the Touch Sensor Block, which is represented by the 1010 0101 symbol. We then need to convert it to text so it can be displayed on the NXT LCD panel.

You may download it here,
Potentio-01.rbt
or write your own.
When I try my potentiometer, I find that the RAW value goes from 0 to 95, pretty close to my predicted range of 0 to 93. So it works! Not bad considering I guessed that the potentiometer sweeps through and angle of  .
.
Determining the Angle of the Potentiometer
Now, let’s convert this RAW value to an angle.
In Extreme NXT, the authors worry about the fact that the resulting relationship is nonlinear with respect to the RAW value. As far as I can see, this isn’t a problem. We simply solve (4) above for the angle  in terms of RAW. We can output the angle if we wish, but here I’ll take it a step further and demonstrate the resulting equation by controlling a motor so that it maintains an angle equal to the angle through which I have rotated the potentiometer.
in terms of RAW. We can output the angle if we wish, but here I’ll take it a step further and demonstrate the resulting equation by controlling a motor so that it maintains an angle equal to the angle through which I have rotated the potentiometer.
I will leave out the algebra. Try it yourself. Solve (4) for angle A:
(5) 
for my potentiometer, this is simply

which is easy to code in NXT-G.
You can download my code here:
Potentio-03.rbt
The motor control is a bit crude, but it works well enough for the demonstration.
Check out the YouTube video to see it in action!
Enjoy!